This guide will show you how to calculate the probability (area under the curve) of a standard normal distribution. It will first show you how to interpret a Standard Normal Distribution Table. It will then show you how to calculate the:
- probability outside two z-values.


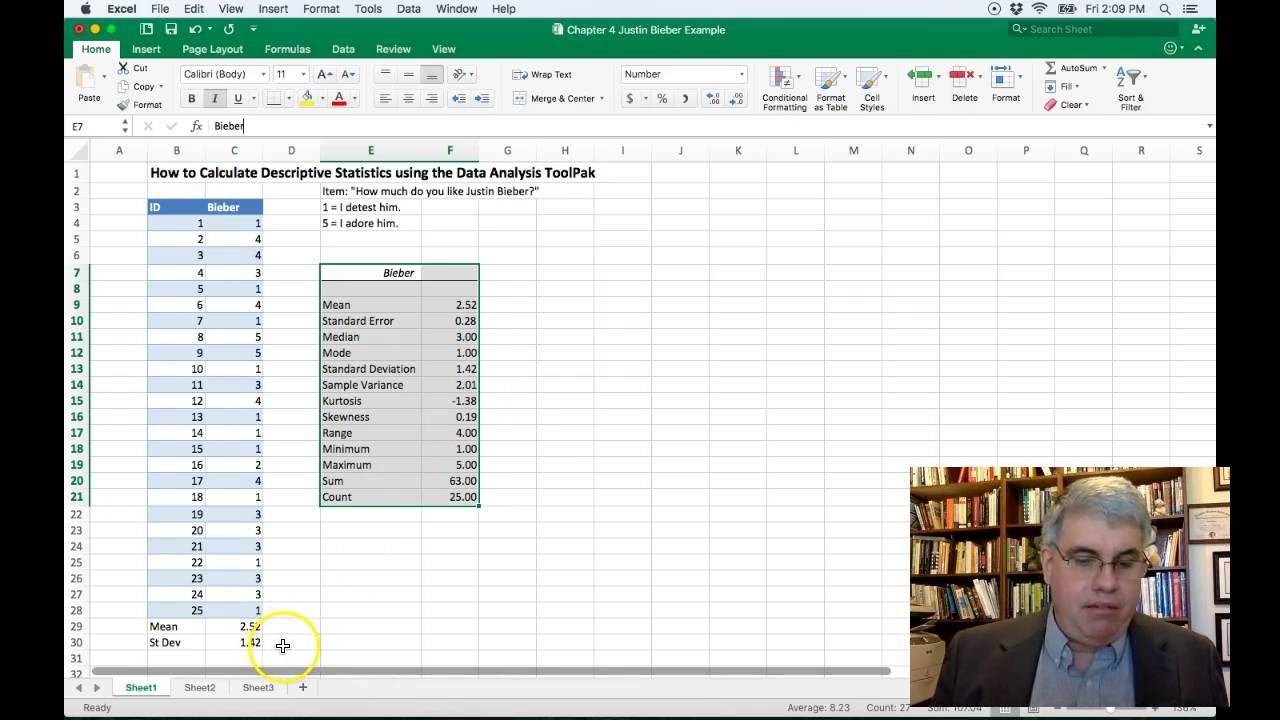
We have a calculator that calculates probabilities based on z-values for all the above situations. In addition, it also outputs all the working to get to the answer, so you know the logic of how to calculate the answer.
How to Use the Standard Normal Distribution Table
The dice probability calculator is a great tool if you want to estimate the dice roll probability over numerous variants. There are may different polyhedral die included, so you can explore the probability of a 20 sided die as well as that of a regular cubic die. This section features several statistics calculators designed to help you solve common statistical calculations. Statistics can be formerly defined as the science that deals with the collection, classification, analysis, and interpretation of numerical facts or data, and that, by use of mathematical theories of probability, imposes order and regularity on aggregates of more or less disparate elements. Data, Surveys, Probability and Statistics at Math is Fun. The mean, or the average of a data set, is one way to measure the center of a numerical data set. The notation for the mean is The formula for the mean is where x represents each of the values in the data set.
The most common form of standard normal distribution table that you see is a table similar to the one below (click image to enlarge):

The Standard Normal Distribution Table
The standard normal distribution table provides the probability that a normally distributed random variable Z, with mean equal to 0 and variance equal to 1, is less than or equal to z. It does this for positive values of z only (i.e., z-values on the right-hand side of the mean). What this means in practice is that if someone asks you to find the probability of a value being less than a specific, positive z-value, you can simply look that value up in the table. We call this area Φ. Thus, for this table, P(Z < a) = Φ(a), where a is positive.
Diagrammatically, the probability of Z less than 'a' being Φ(a), as determined from the standard normal distribution table, is shown below:
Probability less than a z-value
P(Z < –a)
As explained above, the standard normal distribution table only provides the probability for values less than a positive z-value (i.e., z-values on the right-hand side of the mean). So how do we calculate the probability below a negative z-value (as illustrated below)?
We start by remembering that the standard normal distribution has a total area (probability) equal to 1 and it is also symmetrical about the mean. Thus, we can do the following to calculate negative z-values: we need to appreciate that the area under the curve covered by P(Z > a) is the same as the probability less than –a {P(Z < –a)} as illustrated below:
Making this connection is very important because from the standard normal distribution table, we can calculate the probability less than 'a', as 'a' is now a positive value. Imposing P(Z < a) on the above graph is illustrated below:
From the above illustration, and from our knowledge that the area under the standard normal distribution is equal to 1, we can conclude that the two areas add up to 1. We can, therefore, make the following statements:
Φ(a) + Φ(–a) = 1
∴ Φ(–a) = 1 – Φ(a)
Thus, we know that to find a value less than a negative z-value we use the following equation:
Statistical Calculations Definition
Φ(–a) = 1 – Φ(a), e.g. Φ(–1.43) = 1 – Φ(1.43)
